The Mystery of the Cosmos: What Exactly Are We Looking For?
The key to understanding the universe may lie in grasping the ways it replicates itself.
By Andrew StarkOctober 21, 2021
FOR AS LONG as our species can remember, even before Plato and Confucius, we were deploying two pairs of conceptual distinctions to carve up the world and make it understandable: the distinction between parts and wholes, and the distinction between particulars and universals.
A Honda engine is a part that — along with other parts, like the steering wheel, the gears, and the fan belt — composes the “whole” known as a Honda car. That very same engine, meanwhile, is also — along with Toyota engines, General Motors engines, and Ford engines — a particular that embodies or “instantiates” the universal idea of engine-ness.
What happens, then, when we take these two conceptual screens, so helpful for making sense of the perceivable world, and forge out into the heavens and down into the quantum?
Many of the greatest physicists tell us that any fundamental resolution of cosmological mystery will have to be conceptually — mathematically — beautiful. And in terms of theory, as far as we in the early 21st century can tell, any such beautiful resolution will center on a reconciliation of quantum mechanics, which focuses on the universe at a micro level, and the theory of relativity, which describes the universe at a macro level. As Einstein said, speaking of human psychology and not the cosmos, “the only physical theories that we are willing to accept are the beautiful ones.”
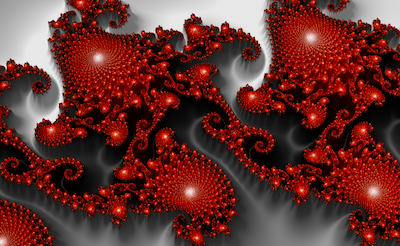
Think of a fern, we are told, with branches sprouting from its main stem. Sprouting from each of those branches there appear yet smaller ones that resemble it, and then sprouting from each of them there are even smaller ones that resemble it — and so on, theoretically, ad infinitum. The branches, then, all vary the same pattern. But they do so at ever tinier scales and shifting positions. Varying the same pattern at ever decreasing magnitudes and altering orientations is, in a basic sense, what a fractal does. For this reason, fractals often are analogized to linguistic dialects, which preserve a language’s structure while varying it across size and location.
Fractals get generated by a recursive algorithm, and each new execution is generated by applying the algorithm in question to the result of the previous one, creating — as these iterations build up and depending on the algorithm’s features — fantastic geometrical figures. And, as the Harvard physicist Nicole Yunger Halpern says, fractals are beginning to provide an “exciting” way of conceptualizing what’s going on at the frontiers of physics. Cosmological systems, scientists are discovering, can “exhibit fractal-like behavior,” Yunger Halpern explains, meaning that “they look very much the same at different spatial and temporal scales.”
In the most exotic specimens, where fabulous spirals, tongues, and brocades begin to appear, the fractal is too smoothly continuous to divide into parts in any meaningful way. It’s much more apt to divide a fractal into components based on each new iteration of the algorithm: first iteration, second iteration, third iteration, and so on. But that means that the “whole” we see is composed not of parts but of particulars: particulars that each instantiate the same universal — that universal, of course, being the algorithm itself. A fractal’s beauty, then, emerges from a crossover of the two ur-distinctions. It emerges from the gorgeous ways in which particulars, not parts, compose a whole.
Physicists find a second source of beauty in the symmetries revealed by and in their calculations. Symmetry varies the same pattern over and over. But not at different scales and orientations, as fractals do. Instead, symmetries vary a pattern through different rotations and reflections.
The physicist Sabine Hossenfelder deploys the analogy of a mandala to give a sense of such reflections and rotations. A mandala takes a pattern and then reflects it so that right becomes left and left becomes right, or rotates it so that up becomes down and down becomes up, over and over again. Both reflective and rotational symmetry form the backbone of some of the most influential theories of modern physics. Paul Dirac’s equations display a form of reflective symmetry, Hossenfelder says, by incorporating particles of antimatter with the “same mass as corresponding particles […] with the opposite charge.” Contemporary string theory embodies a rotational symmetry by which, as Steven Weinberg writes, “when you rotate an object from an ordinary dimension to a quantum dimension […] a particle of force becomes a particle of matter and vice versa.”
Think of a Persian carpet. When you marvel at its symmetry — the same pattern but rotated and reflected in multiple ways — what exactly, in terms of those fundamental concepts, parts and wholes or particulars and universals, are you savoring? Certainly, the parts of the carpet, each containing the exact same pattern, however rotated or reflected, are what, summed together, compose the whole. But they don’t do so in the way a car’s parts — an engine, a fuel tank, a fan belt, and so forth — compose the whole of a car. Unlike the parts of my car, the parts of the carpet are identical, as if my car contained 80 engines, reflected and rotated in relation to each other.
So if the parts of the carpet don’t compose a whole in the typical fashion, maybe it’s more apt to say that the carpet is made up of particulars that each instantiate the same universal, which of course is what a pattern is. But that doesn’t quite work either. For a particular to instantiate a universal, it must embody it in a particular way. A Honda’s engine instantiates the universal “engine” through the particularities of the Honda’s design, while a Toyota’s engine instantiates the universal “engine” through the particularities of a Toyota’s design. But there’s nothing particular, at least in this way, in the “particulars” of the carpet. Each instantiates the universal — the pattern — in exactly the same way, merely rotating or reflecting it. Each part universally, not particularly, embodies the pattern.
Maybe, then, the carpet can best be described as possessing identifiable parts, each of which instantiates the same pure universal, simply rotated and reflected as the case may be. Perhaps the beauty of symmetry, in other words, rests not in the ways in which its parts compose a whole, since they don’t do so the way a car’s parts compose a whole. Nor does it lie in the ways in which it arrays particulars that instantiate a universal since, again, nothing in the carpet particularizes a universal in the way my car’s engine does. Instead, symmetry’s beauty lies in the way it accomplishes a kind of crossover. The beauty of a symmetrical design emerges, it would seem, from the ways its rotationally and reflectively arranged parts each instantiate the same unalloyed universal.
But how is it for the cosmos? Physicists often use the term “symmetry” in an exceedingly broad sense. Symmetry exists whenever some components of a system remain the same as the rest changes, just as the pattern of a carpet remains the same through its various rotations and reflections. An example of such symmetry, for physicists, arises from the basic fact that the laws of physics remain unaltered no matter how much we vary our location in space-time.
As awe-inspiring as that reality might be, it’s not beautiful in the more specific sense of the carpet — in the sense of parts mirroring each other by instantiating the same universal through rotation and reflection. For that, we have to turn to the content of the laws of physics themselves, to the symmetry of Dirac’s equations, for example, or those of string theory. We also find the beauty of parts instantiating the same universal, the same pattern, in Murray Gell-Mann’s discovery that “all the particles could be classified by symmetric patterns known as multiplets,” or Steven Weinberg’s revelation that certain “internal symmetries” between electrons and neutrinos necessitate the existence of the several fields, such as the electromagnetic field, in the Standard Model.
Symmetrical beauty lies not in how various parts compose a whole, nor in how various particulars instantiate a universal. Rather, it lies in how various parts instantiate a universal while rotating or reflecting it. Such features of the cosmos are, for physicists, profoundly beautiful. And they feel profoundly explanatory. Why? Because of the way they ultimately correspond to our understanding of the symmetrical beauty of a snowflake, how the parts of a system instantiate the same universal in mirror images. And the rest of us can, even if from afar, see why.
Beyond fractals and symmetries — and of course, many fractals also display symmetry — physicists find beauty as well in the way in which different aspects of the physical world mathematically “map” each other. The discovery that vastly disparate facets of reality share a common structure or display the same network of relationships — that you can map them onto each other — gives the sense of profound explanatory insight.
Consider, to use a common example, the structural parallels between Joe, John, and Bobby Kennedy and Archie, Peyton, and Eli Manning. The father–elder son– younger son relationships in each family map onto each other exactly, even though the individual elements on either side differ. This kind of sameness between structures is often called “isomorphism,” iso being Greek for “same,” and morph for “shape” or “form.” Because the Kennedys and the Mannings are different individuals, the structures, while isomorphic, are not identical.
When it comes to physics, finding isomorphisms or mutual mappings between otherwise non-identical entities yields deep understanding. “If one has really technically penetrated a subject,” as John von Neumann once said, “things that previously seemed in complete contrast might” reveal themselves as “purely mathematical transformations of each other.” Such “aesthetic” beauty — and hence explanatory satisfaction — can, for example, be found, as the Nobel laureate Subrahmanyan Chandrasekhar says, in the way in which the theory of colliding waves and the theory of black holes map onto each other.
But why is mapping beautiful? And, for those who find beauty explanatorily satisfying, why is isomorphism so satisfyingly explanatory?
Return for a moment to the Kennedys and the Mannings. Each family particularizes a common structure: the structure of father, elder son, and younger son. But though each family might be its own particular, the isomorphism — the common structure — that each instantiates is a kind of whole, not a universal. After all, when it comes to universals, the Kennedys and the Mannings instantiate very different ones. The Kennedys embody the universals of politics, and the Mannings the universals of sports. They are, to use von Neumann’s words, “in complete contrast.” Instead, it’s more apt to say that each of the two particulars instantiates the same whole, if a whole is something greater than the sum of its parts — if it is whatever it is that structures and connects those parts.
Physicists find beauty, as a last example, in equations. Think of E = mc
2
. Energy equals mass times the speed of light squared. Both m and c
2
are “parts,” as the philosopher of science Robert Crease says, of one side of the equation. E, the other side of the equation, is a universal, a property that is instantiated in particles across the cosmos. A useful term for this relationship, in which parts on one side of an equation compose universals on the other side is “translation.” Physicists often employ this term in referring to equations. The metaphor of languages and their translations pervades the philosophical analysis of equations, and it helps explain their beauty. It “could end up being,” as Rodolfo Gambini and Jorge Pullin say, “that string theory and loop quantum gravity both provide quantum theories of gravity cast in different languages.” And the required equations, A. R. P. Rau writes, would then be like “dictionaries allowing us to go from one to another.”
The metaphor of translation, when applied to equations, proves to be an apt one. When a given sentence translates from one language into another, the words in the first do not map onto the words in the second one-to-one. Instead, the words in one language — which are “parts” of speech — together compose a meaning, a universal, in the other. That’s what it means for them to be translated. For example, a string of English words, such as “the moment when a meal is concluded but the people around the table continue to chat,” are all needed, together, to compose the meaning captured by sobremesa in Spanish. Those words are parts of English. The Spanish sobremesa to which they translate is a universal, one we have all experienced in our own particular ways.
The Harvard mathematician Barry Mazur neatly illustrates this translational aspect of equations. He analogizes it to poetry and in so doing highlights its capacity for beauty. “Consider,” Mazur says, “these lines of Yeats: ‘Like a long-legged fly upon the stream / His mind moves upon silence.’”
Here, Mazur observes, “[T]he equation is between something that is concrete/sensual and external (the ‘long-legged fly upon the stream’) and something that might actually be even […] much harder to catch and hold still: a curious interior state.”
In other words, in Yeats’s equation, the stream and the long-legged fly on the one side are parts that together compose the universal, the property of a “curious inner state” — a mind moving upon silence — on the other.
The quest for beauty — and, if beauty is what gives us a sense that we have understood, then the quest for understanding too — ultimately requires us to burst through the ur-categories, the categories through which we see the world as consisting of particulars that instantiate universals and parts that compose wholes. Here, at the precipice of our understanding, we need the ur-categories to switch dance partners. Here, it’s particulars that must pair up with wholes, either composing them as with fractals or instantiating them as with isomorphisms. And it’s parts that must mate with universals — again, either instantiating them as in symmetry or composing them as in equations.
Symmetries get analogized to mirrors, and isomorphisms to maps. And that makes sense; symmetries have to do with one thing repeating itself over and over, while isomorphisms have to do with one thing relating to another. In the same vein, fractals get likened to dialects, and equations to translations. And this, too, makes sense: fractals deal with one thing varying itself over and over, while equations deal with one thing relating to another. Mirrors are to maps what dialects are to translations. Each metaphor contributes to capturing what it is in symmetries, isomorphisms, fractals, and equations that endows them with the potential for transcendent beauty.
Consider the holographic theory that Juan Maldacena, a theoretical physicist at the Institute for Advanced Study, offers to reconcile quantum field theory and relativity. In his principal illustration, Maldacena depicts a disk with various symmetries in its interior, each part instantiating the same universal rotated and reflected. These correspond to the gravitational universe as relativity understands it. But at its edges, the disk turns into fractals, the whole of its circumference being composed of endless particulars of the same algorithm, in various sizes and counter-positions. These represent the quantum. And what’s more, the interior symmetries and the edge fractals can be shown to relate to each other through equations — i.e., translations — insofar as the parts in each compose universals that abide in the other. They also relate as isomorphisms — mutual mappings — in that each, the interior and the edge, is a particular that instantiates the same whole, the same structure. It’s quite magical.
For millennia, we have understood the world through particulars that instantiate universals and parts that compose wholes. Now the mystery of the universe asks that we — the “we” that Einstein referred to, the human community at large — go even further. It beckons us to transcend the limits of our understanding by seeing the cosmos in terms of particulars that instantiate or compose wholes and parts that compose or instantiate universals. That’s what scientific beauty is, as physicists describe it to us. And if the truth must be beautiful, it’s also where the path to ultimate explanation lies.
¤
¤
Featured image: "A 3D version of the Mandelbrot set plot 'Map 44' from the book 'The Beauty of Fractals'" by Duncan Champney is licensed under CC BY-SA 4.0. Image has been cropped.
LARB Contributor
Andrew Stark is a professor of strategic management at the University of Toronto and author of The Consolations of Mortality: Making Sense of Death (Yale University Press, 2016). His work has appeared in The Atlantic, The New York Times, The New York Review of Books, and other publications.
LARB Staff Recommendations
Seeing Time: Einstein’s Theory of Relativity for Beginners
A charming review of a charmingly quirky art-science book about simultaneity (how to see it) and Einstein’s theory of special relativity.
Uncle Miltie’s Never-Ending Show
A reissue of Milton Friedman’s classic work only shows his obsolescence.
Did you know LARB is a reader-supported nonprofit?
LARB publishes daily without a paywall as part of our mission to make rigorous, incisive, and engaging writing on every aspect of literature, culture, and the arts freely accessible to the public. Help us continue this work with your tax-deductible donation today!